How To Find Height Of Cone With Volume And Base Area

In geometry, cone is a solid or hollow object with a round flat base and sides that slope up to a top point. The cone formulas, solved example & step by step calculations may useful for users to understand how the input values are being used in such calculations. Also this featured cone calculator uses the various conversion functions to find its area, volume & slant height in SI or metric or US customary units. A cone is an three-dimensional geometric shape having circular base and only one vertex.

A cone can be formed by the locus of all straight line segments that join the vertex to the base has a rotational symmetry. We can say that a cone have flat circular base and having one side curved surface. The volume and the total surface area of the cone depend upon the radius of base, height and slant height of the cone. To calculate the total surface area of a cone we need radius of circular base and height of cone. Then, it calculates the total surface area of cone using the formula given above and prints the result on screen using printf function. The volume of a cone can be defined as the amount of three dimensional space occupied by the right circular cone or the storage capacity of a right circular cone.

Finding volume of a cone help us to solve many real life problems like, how much ice-cream is required to file an ice-cream cone. To calculate the volume of a right circular cone, we need radius of base height of cone. Volume of a cone is measured in cubic units like meter3, cm3 etc. We come across many geometrical shapes while dealing with geometry. We usually study about two dimensional and three-dimensional figures in school.

They can be drawn on paper, for example – circle, rectangle, square, triangle, polygon, parallelogram etc. The three-dimensional figures cannot be drawn on paper since they have an additional third dimension as height or depth. The examples of 3D shapes are a sphere, hemisphere, cylinder, cone, pyramid, prism etc.

If the cone is right circular the intersection of a plane with the lateral surface is a conic section. In general, however, the base may be any shape and the apex may lie anywhere . Contrasted with right cones are oblique cones, in which the axis passes through the centre of the base non-perpendicularly. If the enclosed points are included in the base, the cone is a solid object; otherwise it is a two-dimensional object in three-dimensional space.

A pyramid consists of three or four triangular lateral surfaces and a three or four sided surface, respectively, at its base. When we calculate the surface area of the pyramid below we take the sum of the areas of the 4 triangles area and the base square. The height of a triangle within a pyramid is called the slant height. In the field of geometry calculations, finding the area, volume & slanting height of a cone is very important to understand a part of basic mathematics.

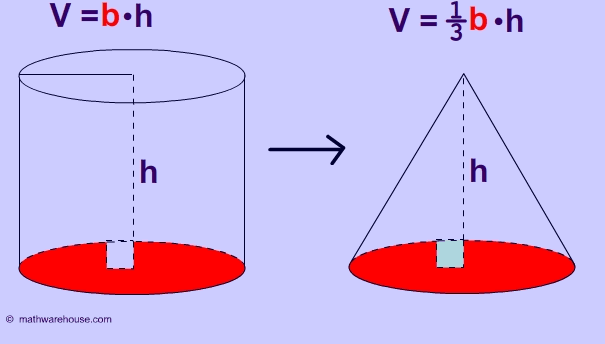
If a cone and cylinder have the same height and base radius, then the volume of cone is equal to one third of that of cylinder. That is, you would need the contents of three cones to fill up this cylinder. The same relationship holds for the volume of a pyramid and that of a prism . A cone is a three dimensional geometric shape with one vertex and a circular base.

The line form the centre of the base to the apex is the perpendicular height. Conical and pyramidal shapes are often used, generally in a truncated form, to store grain and other commodities. Similarly a silo in the form of a cylinder, sometimes with a cone on the bottom, is often used as a place of storage.

It is important to be able to calculate the volume and surface area of these solids. In this module, we will examine how to find the surface area of a cylinder and develop the formulae for the volume and surface area of a pyramid, a cone and a sphere. These solids differ from prisms in that they do not have uniform cross sections.

In geometry, a cone is a 3-dimensional shape with a circular base and a curved surface that tapers from the base to the apex or vertex at the top. In simple words, a cone is a pyramid with a circular base. With the Pythagorean theorem, use the radius and the height to calculate the slant height of the cone, then multiply the slant height by the radius by pi. To that you add the base area of the cone, which is found by multiplying pi by the square of the radius.

The total surface area is found by adding the lateral surface area to the base area. In geometry, a cone is a solid figure with one circular base and a vertex. The height of a cone is the distance between its base and the vertex.The cones that we will look at in this section will always have the height perpendicular to the base. In a cone, the perpendicular length between the vertex of a cone and the center of the circular base is known as the height of a cone.

A cone's slanted lines are the length of a cone along the taper curved surface. All of these parameters are mentioned in the figure above. In the figure, you can see a right circular cone, which has a circular base of radius r and whose axis is perpendicular to the base. The line which connects the vertex of the cone to the centre of the base is the height of the cone. The length at the outer edge of the cone, which connects a vertex to the end of the circular base is the slant height. The figure above also illustrates the terms height and radius for a cone and a cylinder.

The height of the cone is the length h of the straight line from the cone's tip to the center of its circular base. Both ends of a cylinder are circles, each of radius r. The height of the cylinder is the length h between the centers of the two ends. Enter the radius r of the base and height h of the cone as positive real numbers and press "Calculate Area and Volume". The outputs are the lateral surface area \( L_a \) , the base area \( B_a \), the total area \( T_a \), the volume of the cone V and the slant height s.

A cone is a three-dimensional figure with one circular base. A curved surface connects the base and the vertex. Given height and slant height calculate the radius, volume, lateral surface area and total surface area.

Given radius and lateral surface area calculate the height, slant height, volume and total surface area. Given radius and slant height calculate the height, volume, lateral surface area and total surface area. Given radius and height calculate the slant height, volume, lateral surface area and total surface area. The base of a cone is a circle and that is easy to see.

The lateral surface of a cone is a parallelogram with a base that is half the circumference of the cone and with the slant height as the height. This can be a little bit trickier to see, but if you cut the lateral surface of the cone into sections and lay them next to each other it's easily seen. Enter the height h and the slant height s of the cone as positive real numbers such that s greater then h and press "Calculate Area and Volume".

The outputs are the lateral surface area \( L_a \) , the base area \( B_a \), the total area \( T_a \), the volume of the cone V and the radius r. Enter the radius r of the base and the slant height s of the cone as positive real numbers such that s is greater than r and then press "Calculate Area and Volume". The outputs are the lateral surface area \( L_a \) , the base area \( B_a \), the total area \( T_a \), the volume of the cone V and the height h. Let us consider an example where we use the formula for the volume of a cone given a diagram. A right circular cone is a cone where the axis of the cone is the line meeting the vertex to the midpoint of the circular base. That is, the centre point of the circular base is joined with the apex of the cone and it forms a right angle.

A cone is a three-dimensional shape having a circular base and narrowing smoothly to a point above the base. Given slant height, height and radius of a cone, we have to calculate the volume and surface area of the cone. An oblique cone is a cone with an apex that is not aligned above the center of the base.

It "leans" to one side, similarly to the oblique cylinder. The cone volume formula of the oblique cone is the same as for the right one. Given slant height and lateral surface area calculate the radius, height, volume, and total surface area. Given height and volume calculate the radius, slant height, lateral surface area and total surface area. Given radius and total surface area calculate the height, slant height, volume and lateral surface area. Given radius and volume calculate the height, slant height, lateral surface area and total surface area.

Thus we can derive a formula for the volume of a cone of any shaped base if we can do so for some one shaped base. It has a curved surfacewhich tapers (i.e. decreases in size) to a vertex at the top. The height of the cone is the perpendicular distance from the base to the vertex. From which the radius can be estimated if the cone angle is known. The aperture size and the cone angle of the pipette determine its sensitivity to the sample surface.

The sensitivity depends on the ratio of the probe resistance Rp to the probe access resistance Rz. The total surface area of cone is the total area on the outer side of a cuboid. In other words, It is the number of square units that will exactly cover the outer surface of a cone. A cone is a combination of two surfaces, the curved top section with slanted height and the circular base. Hence, The total surface area of cone is sum of area of circular base and area of top curved section.

If a right triangle is rotated about its perpendicular, considering the perpendicular as the axis of rotation, the solid constructed here is the required cone. The surface area generated by the hypotenuse of the triangle is the lateral surface area. The curved surface area is also called the lateral area. Use Reynolds transport theorem to determine the rate at which the cone's volume is increasing when the cone's base radius is ro if its height is h. The following mathematical formulas are used in this cone calculator to find the area, volume & slanting height of a cone.

Since the cross sectional areas are also equal, we can employ Cavalieri's principle and state that the volume of the cone equals the volume of the pyramid. Since the height is the same in both solids, B must equal πr2 for the cone, making the formula for the volume of a cone . We can conduct an experiment to demonstrate that the volume of a cone is actually equal to one-third the volume of a cylinder with the same base and height.

When the water is poured into a cylinder with the same base and height as the cone, the water fills one-third of the cylinder. Sections to learn more about how to find the volume of cones and pyramids and test your understanding. The faces bounding a right pyramid consist of a number of triangles together with the base. To find the surface area, we find the area of each face and add them together. Depending on the information given, it may be necessary to use Pythagoras' Theorem to calculate the height of each triangular face.

If the base of the pyramid is a regular polygon, then the triangular faces will be congruent to each other. The surface area of any right circular cone is the sum of the area of the base and lateral surface area of a cone. The surface area is measured in terms of square units. For a right cone, the lateral surface area is πrl, where r is the radius and l is the slant height.

But the trick is to figure out how to design a 2-D net for the cone. Did you know that the 2-D net for a cone is a sector of a circle? Here, the circle we are talking about has radius s .

But the big question is, how big is the angle of the sector? The amount of the circumference of the sector is the same as the whole circumference of the cone's base, namely, 2𝜋r. Find the total surface area of a cone, whose base radius is 3 cm and the perpendicular height is 4 cm. It consists of a base having the shape of a circle and a curved side ending up in a tip called the apex or vertex. The volumes of a cone and a cylinder are related in the same way as the volumes of a pyramid and a prism are related. If the heights of a cone and a cylinder are equal, then the volume of the cylinder is three times as much as the volume of a cone.

In projective geometry, a cylinder is simply a cone whose apex is at infinity. This is useful in the definition of degenerate conics, which require considering the cylindrical conics. The "base radius" of a circular cone is the radius of its base; often this is simply called the radius of the cone. The aperture of a right circular cone is the maximum angle between two generatrix lines; if the generatrix makes an angle θ to the axis, the aperture is 2θ.

A cone is a three-dimensional geometric shape that tapers smoothly from a flat base to a point called the apex or vertex. This video will demonstrate that the volume of a cone is on-third that of a cylinder with the same base and height. You would need to use calculus for a more rigorous proof.

Find the volume, curved surface, and total surface area of a cone with the given radius 3 and height 4. A Cone is a geometric shape formed by having a circle at one end, usually at a base. It consists of all line segments joining to a single point to every point of a two-dimensional figure.